TS & AP State Syllabus SSC 10th Class Maths Solutions 3rd Lesson Polynomials Exercise 3.2

TS & AP State Syllabus SSC 10th Class Maths Solutions 3rd Lesson Polynomials Exercise 3.2

10th Class Maths 3rd Lesson Polynomials Ex 3.2 Textbook Questions and Answers 

Question 1.
The graphs of y = p(x) are given in the figure below, for some polynomials p(x). In each case, find the number of zeroes of p(x).

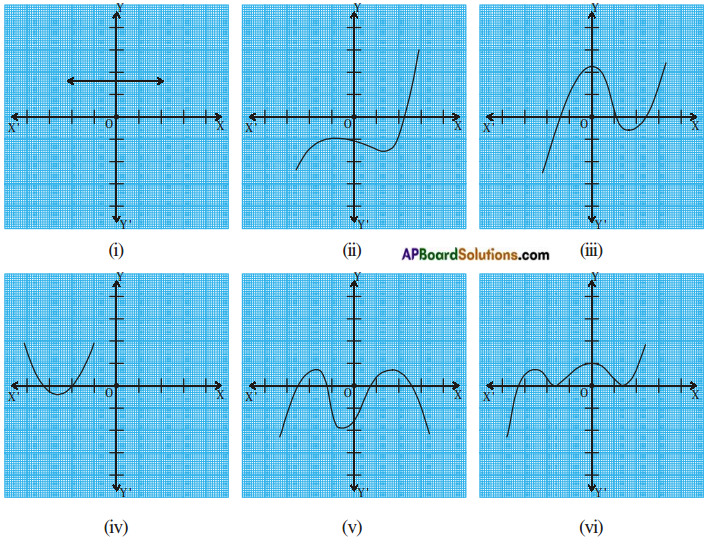
Answer:
i) There are no zeroes as the graph does not intersect the X – axis.
ii) The number of zeroes is one as the graph intersects the X – axis at one point only.
iii) The number of zeroes is three as the graph intersects the X – axis at three points.
iv) The number of zeroes is two as the graph intersects the X – axis at two points.
v) The number of zeroes is four as the graph intersects the X – axis at four points.
vi) The number of zeroes is three as the graph intersects the X – axis at three points.

Question 2.
Find the zeroes of the given polynomials,
(i) p(x) = 3x
(ii) p(x) = x2 + 5x + 6
(iii) p(x) = (x + 2) (x + 3)
(iv) p(x) = x4 – 16
Answer:
i) Given p(x) = 3x
Let p(x) = 0
So, 3x = 0
x =

03

= 0,
Zeroes of p(x) = 3x is zero.
∴ No. of zeroes is one.

ii) Given p(x) = x2 + 5x + 6 is a quadratic polynomial.
It has atmost two zeroes.
To find zeroes, let p(x) = 0
⇒ x2 + 5x + 6 = 0
⇒ x2 + 3x + 2x + 6 = 0
⇒ x(x + 3) + 2 (x + 3) = 0
⇒ (x + 3) (x + 2) = 0
⇒ x + 3 = 0 or x + 2 = 0
⇒ x = -3 or x = -2
Therefore the zeroes of the polynomial are -3 and -2.

iii) Given p(x) = (x + 2) (x + 3)
It is a quadratic polynomial.
It has atmost two zeroes.
Let p(x) = 0
⇒ (x + 2) (x + 3) = 0
⇒ (x + 2) = 0 or (x + 3) = 0
⇒ x = -2 or x = -3
Therefore the zeroes of the polynomial are -2 and – 3.

iv) Given p(x) = x4 – 16 is a biquadratic polynomial. It has atmost two zeroes.
Let p(x) = 0
⇒ x4 – 16 = 0
⇒ (x2)2 – 42 = 0
⇒ (x2 – 4) (x2 + 4) = 0
⇒ (x + 2) (x – 2) (x2 + 4) = 0
⇒ (x + 2) = 0 or (x – 2) = 0 or (x2 + 4) = 0
⇒ x = -2 (or) x = 2 (or) x2 = -4
Therefore the zeroes of the polynomial are 2, – 2, we do not consider √-4 since it is not real.

Question 3.
Draw the graphs of the given polynomial and find the zeroes. Justify the answers,
i) p(x) = x2 – x – 12
ii) p(x) = x2 – 6x + 9
iii) p(x) = x2 – 4x + 5
iv) p(x) = x2 + 3x – 4
v) p(x) = x2 – 1
Answer:
i) Given polynomial p(x) = x2 – x – 12.
List of values of p(x):

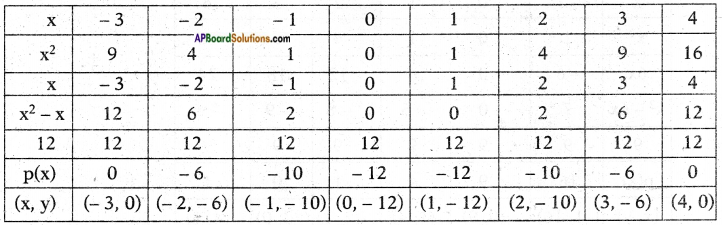
Now, let’s locate the points listed above on a graph paper and draw the graph.

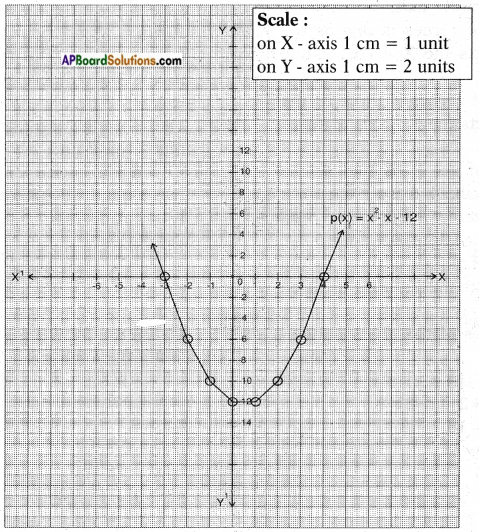
Result: We observe that the graph cuts the X – axis at (-3, 0) and (4, 0).
So, the zeroes of the polynomial are -3 and 4.
Justification:
Given p(x) = x2 – x – 12 = 0
⇒ x2 – 4x + 3x – 12 = 0
⇒ x(x – 4) + 3(x – 4) = 0
⇒ (x – 4) (x + 3) = 0
⇒ x – 4 = 0 and x + 3 = 0
x = 4 and x = – 3

ii) Given polynomial p(x) = x2 – 6x + 9
List of values of p(x):

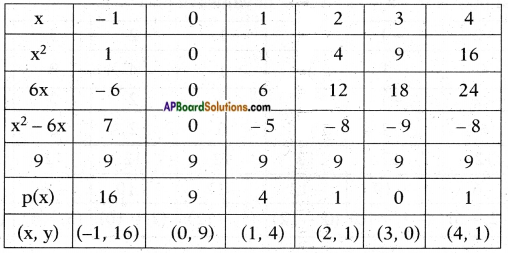
Now, let’s locate the points listed above on a graph paper and draw the graph.

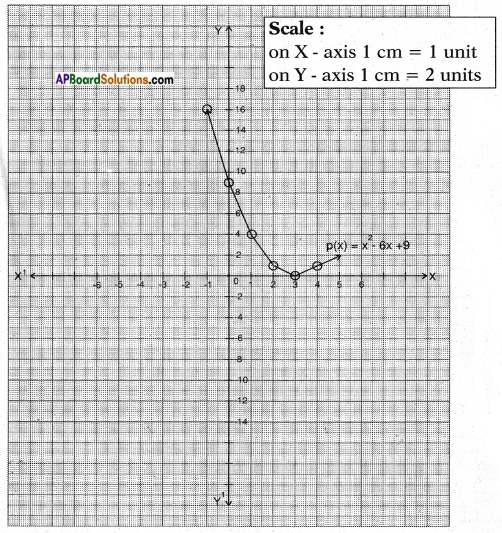
Result: We observe that the graph cuts the X – axis at (3, 0).
So, the zeroes of the given polynomial are same i.e., 3.
Justification:
Given p(x) = x2 – 6x + 9
⇒ x2 – 3x – 3x + 9 = 0
⇒ x(x – 3) – 3(x – 3) = 0
⇒ (x – 3) (x – 3) = 0
⇒ x – 3 = 0 and x – 3 = 0
x = 3 and x = 3

iii) Given polynomial p(x) = x2 – 4x + 5
List of values of p(x):

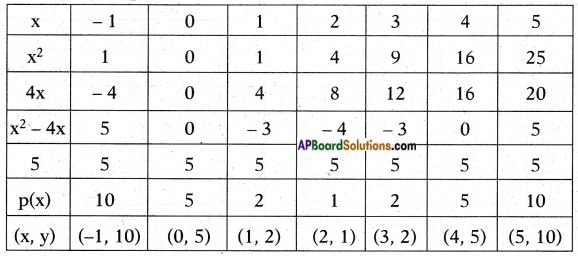
Now, let’s locate the points listed above on a graph paper and draw the graph.

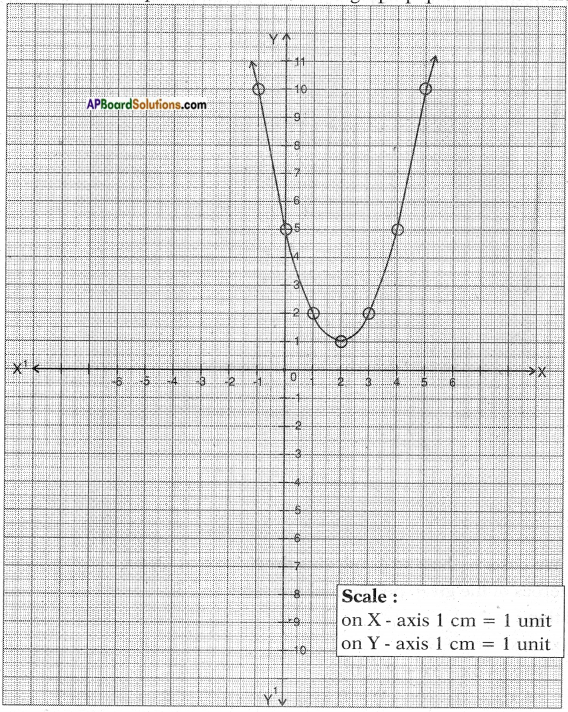
Result: We observe that the graph does not cut the X – axis at any point.
So, the quadratic polynomial p(x) has no zeroes.
Justification: For the given p(x) = x2 – 4x + 5 not possible to split in factors.

iv) Given polynomial p(x) = x2 + 3x – 4.
List of values of p(x):

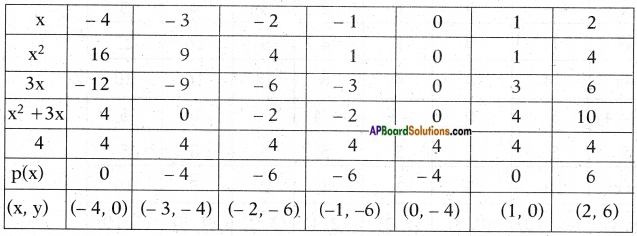
Now, let’s locate the points listed above on a graph paper and draw the graph.

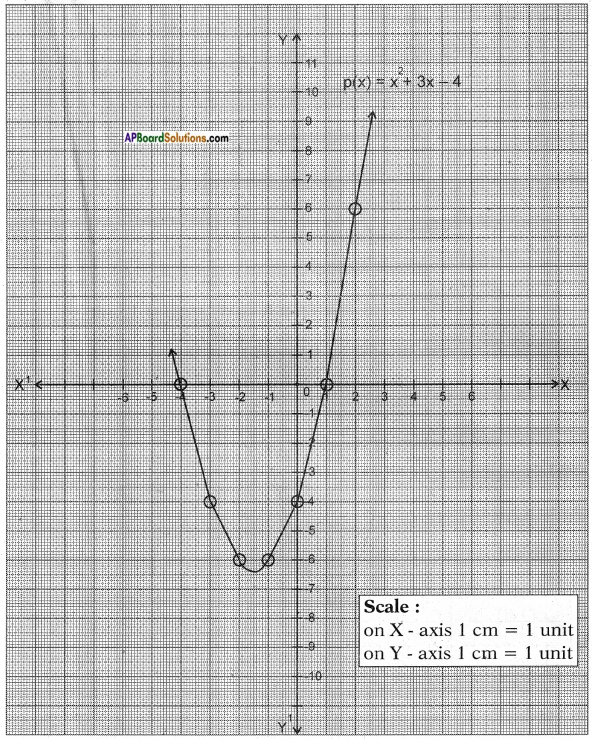
Result: We observe that the graph cuts the X – axis at (-4, 0) and (1, 0).
So, the zeroes of the polynomial are -4 and 1.
Justification:
Given p(x) = x2 + 3x – 4 = 0
⇒ x2 + 4x – x – 4 = 0
⇒ x(x + 4)- 1(x + 4) = 0
⇒ (x + 4) (x – 1) = 0
⇒ x + 4 = 0 and x – 1 = 0
x = – 4 and x = 1

v) Given polynomial p(x) = x2 – 1
List of values of p(x):

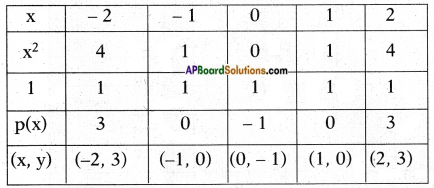
Now, let’s locate the points listed above on a graph paper and draw the graph.

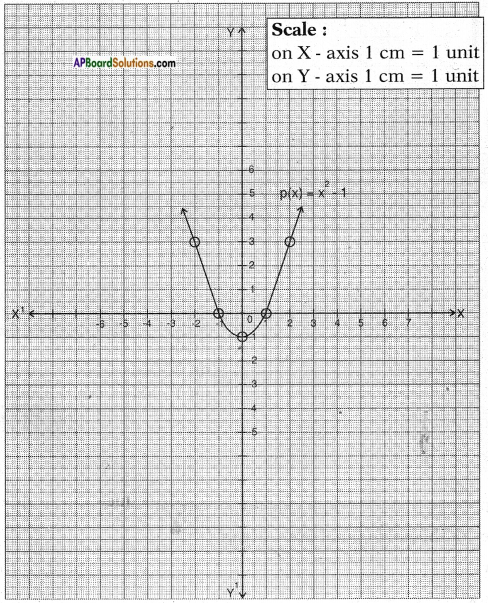
Result: We observe that the graph cuts the X – axis at (-1, 0) and (1,0).
So, the zeroes of the polynomial are – 1 and 1.
Justification:
Given p(x) = x2 – 1 = 0
⇒ p(x) = (x + 1) (x – 1) = 0 [∵ a2 – b2 = (a + b) (a – b)]
⇒ x + 1 = 0 and x – 1 = 0
x = -1 and x = 1

Question 4.
Why are

14

and -1 zeroes of the polynomial p(x) = 4x2 + 3x – 1 ?
Answer:
Given polynomial p(x) = 4x2 + 3x – 1
Given zeroes are

14

and -1
Let x =

Let x = -1
⇒ p(-1) = 4(-1)2 + 3(-1)-1 = 4 – 3 – 1 = 4 – 4 = 0
∴ P(

14

) = 0 and p(-1) = 0
So these values are zeroes of the polynomial p(x).